Dispersive distortions of signals in an analog fiber-optic link with direct intensity modulation
V.V.Shcherbakov1, A.F.Solodkov1, A.A.Zadernovsky2
1JSC “Center VOSPI”, 3 Vvedenskogo St., 117342 Moscow, Russia
2Department of physics, Moscow Technological University, MIREA, 78 Vernadskogo Аv., 119454 Moscow, Russia
zadernovsky@mirea.ru
This work was partially supported by Ministry of Education and Science of the Russian Federation.
I. INTRODUCTION
Fiber-optic links are widely used in various areas of science and technology. In the field of telecommunications, digital data transmission systems are mostly employed. However, the simplest analog systems with direct RF/microwave intensity modulation at the input (by modulating the injection current of semiconductor laser), transportation of the signal of modulation through an optical fiber and, finally, direct detection of photocurrent at the output are in demand for a variety of applications. Analog fiber-optic links are often the only option for some applications, and, at the same time, they are a reliable and cost-effective solution.
II. EXPERIMENTAL AND THEORETICAL RESULTS
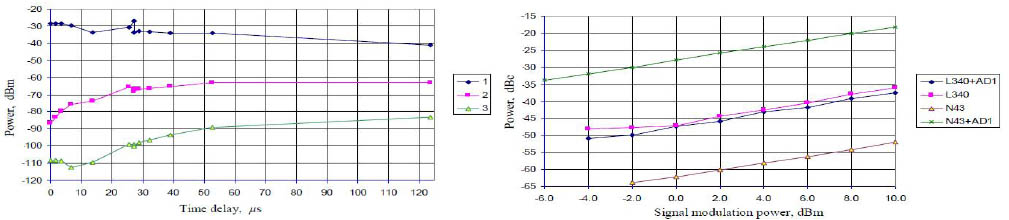
Fig. 1 shows the curves for the output-to-input power ratio of the electrical signal (expressed in dB) versus the modulation frequency fm for several segments of a single-mode fiber of different length L with the dispersion coefficient D = 16 ps/(nm km). We use a single-frequency DFB InGaAsP laser with the emission wavelength l = 1550 nm. Nonlinear distortions (Fig. 2a) manifest themselves in generation of the harmonics that were not present in the original signal. As it can be seen from Fig. 2b, such distortions occur even at low laser light
intensities.
Theoretical interpretation includes the frequency chirping of laser radiation and the group velocity dispersion of electromagnetic waves in optical fiber. The frequencies of the power extrema in Fig. 1 were found to be equal
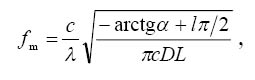 |
(1) |
where odd integers l give the minima, whereas even integers l give the maxima and α is the chirp parameter, also known as Henry factor. The values of the signal power extrema are related with the laser-specific parameter k which is referred to as the adiabatic chirp coefficient. Laser vendors usually do not specify the chirp parameters. Applying the data presented in [1] (α=2.8±0.2, k = (11.4 ± 0.5) c-1 mW-1) we obtain a good agreement between our experimental and theoretical results.
The nonlinear distortions shown in Fig. 2 are due to the group velocity dispersion of electromagnetic waves. Such distortions are not associated with the power of transmitted signals and occur even at low laser light intensities. We have found that signals with the frequencies
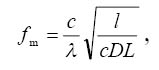 |
(2) |
where l=1, 2, 3... are not subjected to the harmonic distortions.
REFERENCES
[1] A. Villafranca, J. Lasobras and I. Garcés, “Precise characterization of the frequency chirp in directly modulated DFB lasers” Proceedings of 6th Spanish Conference on Electronic Devices 2007, pp. 173-176.
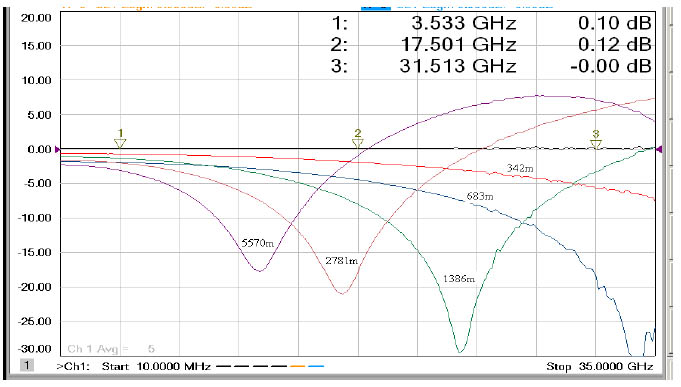 |
| Fig. 1. Output-to-input power ratio of the electrical signal (expressed in dB) versus the modulation frequency for several segments of a single-mode fiber. |
|
|
|
|
a) |
b) |
|
Fig. 2. a) Power of harmonics at the output of a fiber versus the time delay: b) Relative power of the second harmonic (harmonic-to-carrier power ratio) versus the power of signal for several laser sources. Signal frequency is 8.5 GHz. |
|
Мы будем рады ответить на них!